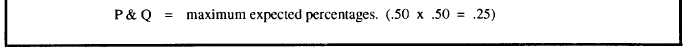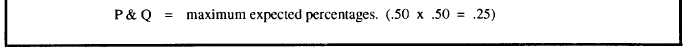l
Figure 9-2.—Sample size increase vs. accuracy.
What are the time and cost constraints? Do
you have the time, manpower and money to
accomplish whatever survey project you are
attempting? This is important. Set deadlines and
give specific job assignments or else piles of
survey junk may end up stashed in someone’s
desk drawer.
SAMPLE SELECTION
How large should a sample be from any given
population? This question takes us into the mathematics
of probability. Do not worry, you will not have to
understand the statistics behind national surveys
produced by the likes of George Gallup. But perhaps a
quote from Mr. Gallup might help put the question of
sample size in perspective. “Both experience and
statistical theory point to the conclusion that no major
poll in the history of this country ever went wrong
because too few persons were reached.”
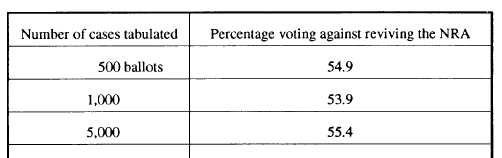
Gallup conducted a number of experiments on the
effects of sample size. In 1936, he used 30,000 ballots
to ask the question: “Would you like to see the National
Recovery Act (NRA) revived?” The first 500 ballots
showed a “no” vote of 54.9 percent. The complete
sample of 30,000 ballots returned a “no” vote of 55.5
percent. In other words, the addition of 29,500 ballots
to the first 500 ballots only made a difference of 0.6
percent. (See fig. 9-2.)
Through the mathematics of probability, we know
there is a real but unknown distribution of all possible
answers to a question. If we then know that our sample
is random (meaning that every person in our audience
is just as likely to get a survey as any other person) and
that our techniques are capable of obtaining a reliable
response (without bias) from each person, we will be
able to tell how representative the responses are.
For the purposes of this chapter, the Sociology
Department at the University of West Florida supplied
a quick and easy formula often used in social science
research. It is shown in figure 9-3.
Figure 9-3.—Sample size formula.
9-6